Kelly Criterion Formula
- Kelly Criterion Formula Python
- Kelly Criterion Formula For Horse Racing
- Kelly Criterion Formula For Excel
- Kelly Criterion Formula For Excel
On this page you'll find a Kelly Criterion Bet Calculator. Enter your assumptions on
- Probability of winning
- Odds and payouts
- Your current bankroll
- Any adjustments you want to make to be conservative
We automatically calculate your ideal bet size with the Kelly Criterion and your assumptions.
Apr 09, 2019 The Kelly Criterion is a formula which accepts known probabilities and payoffs as inputs and outputs the proportion of total wealth to bet in order to achieve the maximum growth rate. The Kelly Criterion is a formula to determine the proper size of a bet with known odds and a definite payout. With hand waving and basic math you can also use it to help guide your investment decisions. It's most useful to determine the size of a position you should take. Using the Kelly Calculator. The Kelly Criterion is well-known among gamblers as a way to decide how much to bet when the odds are in your favor. Most only know a simplified version. Most only know a simplified version. We will show why that holds, but our main goal is to explain the full version.
The Kelly Criterion Bet Calculator

Practical Application of the Kelly Criterion To Betting Strategies
The Kelly Criterion is a formula to determine the proper size of a bet with known odds and a definite payout. With hand waving and basic math you can also use it to help guide your investment decisions.
It's most useful to determine the size of a position you should take.
Using the Kelly Calculator
The Kelly Criterion bet calculator above comes pre-filled with the simplest example: a game of coin flipping stacked in your favor.
- The casino is willing to pay 2 to 1 on any bet you make.
- Your odds of winning any one flip are 50/50.
- Therefore, your probability is .5... 50%.
- Your 'odds offered' are '2 to 1' (so enter 2).
- You have $1,000 with you.
Hit calculate, and see that you should definitely take the bet. Your optimal bet size is 25% of your bankroll.
(Now, find a casino stupid enough to offer those odds!)
Of course, you can see practical the practical value of Kelly betting when it comes to things with discrete results and obvious probabilities - say pot odds in a poker hand. Your mileage may vary.
What do you think about simple Kelly betting? Even though it is designed to never let you go bankrupt, Kelly still allows wild volatility swings.
Do you prefer another strategy? Perhaps half or quarter Kelly methods?
The Kelly Criterion has come to be accepted as one of the most useful staking methods for sharp bettors. While most of us think we have an understanding of the Kelly Criterion and how it works, this is merely a simplified version of the formula. Our latest Guest Contributor has provided an in-depth explanation of the “real” Kelly Criterion. Read on to learn more.
Anyone who is unfamiliar with how the Kelly Criterion can be used to determine optimal bet sizes should read Dominic Cortis’ article on how to use the Kelly Criterion for betting. This approach works well in most cases, however, there are some situations where the Kelly Criterion formula can give some head-scratching results.
Using the examples below, we can examine the potential flaws in using a simplified Kelly Criterion formula.
Example #1 - A soccer game where both a visitor win and draw outcome provide the bettor with an edge:
The Kelly formula would suggest staking 2.5% of bankroll on both the visitor win and the draw, staking a total of 5% of bankroll. Looking at the Handicap odds for the same soccer game changes how we might view the use of the Kelly Criterion.
Example #1A - The same soccer game in example 1 re-stated as a Handicap line:
A bet on the visitor +0.5 at odds of 2.50 is the equivalent to betting half the amount on both the visitor win and draw (both at odds of 5.00). So why does the Kelly formula give a different answer?
The answer is that the formula commonly known as the Kelly Criterion is not the real Kelly Criterion - it is a simplified form that works when there is only one bet at a time.
How to use the “real” or generalised Kelly Criterion
Below is an explanation of how to apply the generalised Kelly Criterion to betting:
Step - 1: List all possible outcomes for the entire set of bets.
Step - 2: Calculate the probability of each outcome.
Step - 3: For each possible outcome, calculate the ending bankroll for that outcome (starting bankroll plus all wins minus all losses). Leave the bet amounts as variables.
Step - 4: Take the logarithm of each ending bankroll from step 3.
Step - 5: Calculate the weighted average of the logarithms from step 4, weighted by the probabilities from step 2. Call this the “objective”.
Step - 6: Find the set of bets that maximises the objective from step 5. These are the optimal bets according to the Kelly Criterion.
In order to find the set of bets that maximises the objective, simply use Microsoft Excel’s built-in “solver” module (see below) - this takes care of the complexities of advanced calculus and eliminates a tedious trial-and-error approach.
The result from using these six steps is as follows:
Note that this is identical to the result in Example #1A, where the simplified version of the Kelly Criterion does work. By making two mutually exclusive bets on the same game, the two bets act as a partial hedge for each other – reducing the overall level of risk, which Kelly rewards by increasing the bet amount (compared to the calculation in Example #1).
Additional uses from the generalised Kelly Criterion
We have already seen how this generalised Kelly Criterion can produce completely different results than the simplified Kelly formula that most bettors will use when there are multiple edges in the same game.
There are, of course, occasions when you might have multiple edges on different games, all taking place at the same time. The example below is one such situation:
Example #2 - Betting with an edge on four separate games that are all taking place at the same time.
Kelly Criterion Formula Python
Now while each of these bets make sense individually, using the simplified Kelly Criterion would result in staking 110% of bankroll - something that clearly doesn’t make sense. However, by applying the six steps stated above, we can see how the generalised Kelly Criterion produces a different set of results.
Because the four games are independent, the probability of each outcome can be calculated as the product of the probabilities of each game; for example, the first row probability would be calculated as:
In additional to calculating the optimal staking amount for a bet with multiple edges, the generalised Kelly Criterion can also be used when bettors have a viable hedging opportunity.
Example #3 - Hedging Garbine Muguruza to win Wimbledon in 2015.
Using the 2015 Wimbledon tournament example previously used in this hedging article, we can see how the generalised Kelly Criterion should be applied to a hedging opportunity.
If you had €1,000 starting bankroll, and you bet €10 on Muguruza at 41.00 to win Wimbledon 2015 outright, you would have to decide how much to hedge on Williams at 1.85 in the final. Let’s assume that the odds in the final are efficient, that is, they accurately reflect each outcome’s probability so that there is no edge on either side.
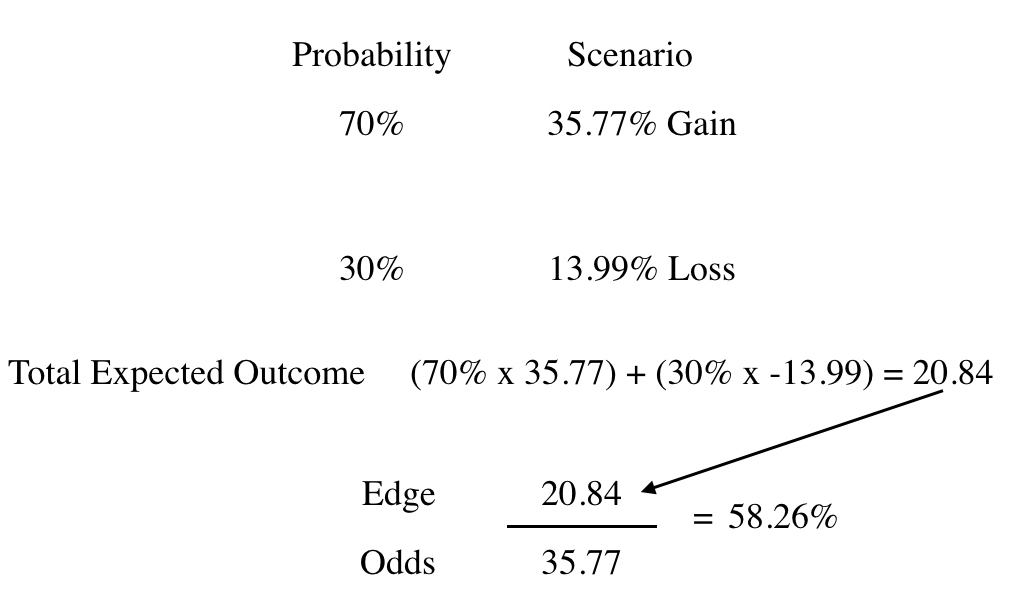
The more commonly used simplified Kelly formula would provide the following results in the scenario:
However, applying the generalised Kelly procedure as stated above yields the results below:
Using this method shows that optimal strategy would be to bet €183.41 on Williams at 1.85 to beat Murguruza in the final and win the tournament - this will hedge most, but not all, of your open position on Murguruza to win the tournament.
Taking the exponential of the objective gives an interesting number, called the “certainty equivalent”. In Example #3 above, the certainty equivalent is exp(7.072341) = 1,178.90. This means that, from a Kelly Criterion perspective, the bettor would be indifferent between having the listed set of bets and having €1,178.90 in risk-free cash.
If we remove the hedge bet, we are left with the following:
So, the effect of the hedge bet is to raise the certainty equivalent from €1,166.87 to €1,178.90. So even though the hedge bet itself has a negative expected value, the resulting reduction in risk is so beneficial that from a Kelly perspective, it has created added value that’s equivalent to €12.03 in cash.
Some other applications of Generalised Kelly
Kelly Criterion Formula For Horse Racing
- Finding optimal bet sizes for a set of “round robin” combinations of parlays or teasers;
- Finding optimal bet sizes for a set of futures bets on several different teams to win the same division or championship;
- Deciding between different ways to hedge an existing bet (money line, spread, buying/selling points), especially if some options result in a “middle” opportunity;
- Figuring out how much to add to, or exit from, an existing position after a line move.
Kelly Criterion Formula For Excel
Use the real Kelly Criterion to empower your betting - get the lowest margins and highest limits online at Pinnacle.
Kelly Criterion Formula For Excel
This Guest Contribution was made by on of our Twitter followers - @PlusEVAnalytics. If you would like to make your own contribution, contact us on Twitter or email us.